Graph Symmetric To X Axis

Learning Outcomes

• Determine amplitude, period, phase shift, and vertical shift of a sine or cosine graph from its equation.
• Graph variations of y=cos x and y=sin x .
• Make up one's mind a office formula that would have a given sinusoidal graph.
• Determine functions that model round and periodic movement.

Graph variations of  y=sin( ten )  and  y=cos( 10 )

Recall that the sine and cosine functions relate real number values to the 10– and y-coordinates of a indicate on the unit circumvolve. And so what do they wait like on a graph on a coordinate plane? Let's get-go with the sine function. We can create a table of values and use them to sketch a graph. The table below lists some of the values for the sine function on a unit of measurement circle.

ten	0	[latex]\frac{\pi}{6}[/latex]	[latex]\frac{\pi}{4}[/latex]	[latex]\frac{\pi}{3}[/latex]	[latex]\frac{\pi}{ii}[/latex]	[latex]\frac{two\pi}{3}[/latex]	[latex]\frac{three\pi}{four}[/latex]	[latex]\frac{5\pi}{half dozen}[/latex]	[latex]\pi[/latex]
[latex]\sin(x)[/latex]	0	[latex]\frac{1}{ii}[/latex]	[latex]\frac{\sqrt{2}}{two}[/latex]	[latex]\frac{\sqrt{3}}{2}[/latex]	1	[latex]\frac{\sqrt{iii}}{2}[/latex]	[latex]\frac{\sqrt{ii}}{2}[/latex]	[latex]\frac{1}{2}[/latex]	0

Plotting the points from the table and standing forth the x-axis gives the shape of the sine function. Meet Figure two.

Figure 2. The sine function

Notice how the sine values are positive between 0 and π, which represent to the values of the sine function in quadrants I and Two on the unit circle, and the sine values are negative betwixt π and 2π, which correspond to the values of the sine office in quadrants Iii and Four on the unit circle. Run into Figure 3.

Figure iii. Plotting values of the sine function

Now permit's take a like await at the cosine function. Again, nosotros can create a tabular array of values and use them to sketch a graph. The table below lists some of the values for the cosine office on a unit circle.

x	0	[latex]\frac{\pi}{6}[/latex]	[latex]\frac{\pi}{4}[/latex]	[latex]\frac{\pi}{3}[/latex]	[latex]\frac{\pi}{2}[/latex]	[latex]\frac{2\pi}{3}[/latex]	[latex]\frac{3\pi}{four}[/latex]	[latex]\frac{5\pi}{6}[/latex]	[latex]\pi[/latex]
[latex]\cos(x)[/latex]	one	[latex]\frac{\sqrt{3}}{2}[/latex]	[latex]\frac{\sqrt{2}}{2}[/latex]	[latex]\frac{ane}{ii}[/latex]	0	[latex]-\frac{1}{2}[/latex]	[latex]-\frac{\sqrt{2}}{two}[/latex]	[latex]-\frac{\sqrt{iii}}{2}[/latex]	−1

As with the sine office, we tin plots points to create a graph of the cosine role as in Effigy four.

Figure iv. The cosine function

Because we can evaluate the sine and cosine of any real number, both of these functions are defined for all real numbers. Past thinking of the sine and cosine values as coordinates of points on a unit circle, it becomes clear that the range of both functions must be the interval [−i,1].

In both graphs, the shape of the graph repeats later 2π,which means the functions are periodic with a period of [latex]2π[/latex]. A periodic function is a function for which a specific horizontal shift, P, results in a function equal to the original function: [latex]f (ten + P) = f(x)[/latex] for all values of x in the domain of f. When this occurs, we call the smallest such horizontal shift with [latex]P > 0[/latex] the period of the function. Figure 5 shows several periods of the sine and cosine functions.

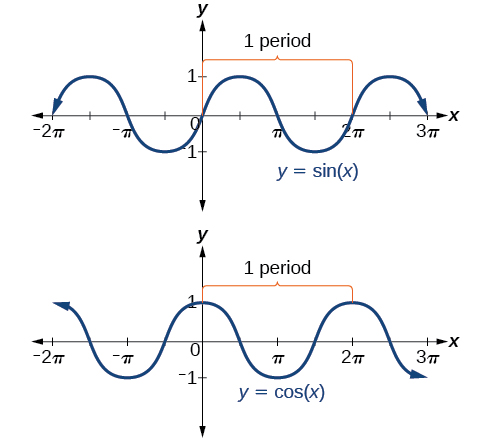
Effigy 5

Looking again at the sine and cosine functions on a domain centered at the y-axis helps reveal symmetries. As we can encounter in Effigy 6, the sine part is symmetric virtually the origin. Call up from The Other Trigonometric Functions that we determined from the unit circle that the sine part is an odd function because [latex]\sin(−x)=−\sin ten[/latex]. At present nosotros can conspicuously run into this holding from the graph.

Figure 6. Odd symmetry of the sine function

Figure seven shows that the cosine function is symmetric about the y-axis. Once again, we adamant that the cosine function is an even function. Now we can meet from the graph that [latex]\cos(−x)=\cos 10[/latex].

Figure 7. Even symmetry of the cosine function

A General Note: Characteristics of Sine and Cosine Functions

The sine and cosine functions have several distinct characteristics:

• They are periodic functions with a period of 2π.
• The domain of each role is [latex]\left(-\infty,\infty\right)[/latex] and the range is [latex]\left[−1,1\right][/latex].
• The graph of [latex]y=\sin x[/latex] is symmetric nearly the origin, because it is an odd function.
• The graph of [latex]y=\cos x[/latex] is symmetric nigh the y-axis, because it is an even function.

Investigating Sinusoidal Functions

As we tin can see, sine and cosine functions have a regular menstruum and range. If we watch ocean waves or ripples on a pond, nosotros will run across that they resemble the sine or cosine functions. All the same, they are non necessarily identical. Some are taller or longer than others. A function that has the same full general shape equally a sine or cosine role is known as a sinusoidal function. The full general forms of sinusoidal functions are

[latex]y = A\sin (Bx−C) + D[/latex]

and

[latex]y = A\cos (Bx−C) + D[/latex]

Determining the Period of Sinusoidal Functions

Looking at the forms of sinusoidal functions, nosotros can see that they are transformations of the sine and cosine functions. We can utilize what we know most transformations to determine the period.

In the general formula, B is related to the menstruum by [latex]P=\frac{2π}{|B|}[/latex]. If [latex]|B| > 1[/latex], then the menses is less than [latex]2π[/latex] and the function undergoes a horizontal compression, whereas if [latex]|B| < ane[/latex], so the period is greater than [latex]2π[/latex] and the function undergoes a horizontal stretch. For case, [latex]f(ten) = \sin(x), B= 1[/latex], so the period is [latex]2π[/latex], which we knew. If [latex]f(x) =\sin (2x)[/latex], and then [latex]B= 2[/latex], so the period is [latex]π[/latex] and the graph is compressed. If [latex]f(10) = \sin\left(\frac{x}{ii} \right)[/latex], then [latex]B=\frac{1}{two}[/latex], so the menstruation is [latex]4π[/latex] and the graph is stretched. Notice in Figure 8 how the period is indirectly related to [latex]|B|[/latex].

Figure 8

A Full general Note: Menses of Sinusoidal Functions

If we let C = 0 and D = 0 in the full general class equations of the sine and cosine functions, we obtain the forms

[latex]y=A\sin\left(Bx\correct)[/latex]

[latex]y=A\cos\left(Bx\right)[/latex]

The period is [latex]\frac{2π}{|B|}[/latex].

Example 1: Identifying the Period of a Sine or Cosine Part

Determine the period of the function [latex]f(ten) = \sin\left(\frac{π}{6}x\right)[/latex].

Try It

Determine the period of the part [latex]g(x)=\cos\left(\frac{x}{three}\right)[/latex].

Show Solution

[latex]six \pi[/latex]

Determining Aamplitude

Returning to the general formula for a sinusoidal role, we have analyzed how the variable B relates to the catamenia. Now permit's plough to the variable A so nosotros can analyze how it is related to the amplitude, or greatest altitude from residual. A represents the vertical stretch factor, and its absolute value |A| is the aamplitude. The local maxima volition be a distance |A| above the vertical midline of the graph, which is the line x= D; because D = 0 in this case, the midline is the x-axis. The local minima will be the aforementioned distance below the midline. If |A| > 1, the function is stretched. For case, the amplitude of [latex]f(x)=four\sin\left(x\correct)[/latex] is twice the aamplitude of

[latex]f(ten)=2\sin\left(x\right)[/latex]

If [latex]|A| < 1[/latex], the office is compressed. Figure 9 compares several sine functions with different amplitudes.

Effigy 9

A General Note: Amplitude of Sinusoidal Functions

If we let C= 0 and D = 0 in the general class equations of the sine and cosine functions, we obtain the forms

[latex]y=A\sin(Bx)[/latex] and [latex]y=A\cos(Bx)[/latex]

The amplitude is A, and the vertical meridian from the midline is |A|. In addition, notice in the example that

[latex]|A|=\text{amplitude}=\frac{ane}{2}|\text{maximum}−\text{minimum}|[/latex]

Case two: Identifying the Amplitude of a Sine or Cosine Function

What is the amplitude of the sinusoidal function [latex]f(x)=−4\sin(x)[/latex]? Is the function stretched or compressed vertically?

Try Information technology

What is the amplitude of the sinusoidal part [latex]f(x)=12\sin (x)[/latex]? Is the function stretched or compressed vertically?

Evidence Solution

[latex]\frac{1}{two}[/latex] compressed

Analyzing Graphs of Variations of y = sin 10 and y = cos x

Now that we empathise how A and B relate to the full general form equation for the sine and cosine functions, we will explore the variables Cand D. Think the general course:

[latex]y = A \sin(Bx−C)+D[/latex] and [latex]y=A\cos(Bx−C)+D[/latex]

or

[latex]y=A\sin(B(10−\frac{C}{B}))+D[/latex] and [latex]y=A\cos(B(x−\frac{C}{B}))+D[/latex]

The value [latex]\frac{C}{B}[/latex] for a sinusoidal function is called the phase shift, or the horizontal displacement of the basic sine or cosine role. If C > 0, the graph shifts to the right. If C < 0,the graph shifts to the left. The greater the value of |C|, the more the graph is shifted. Effigy 11 shows that the graph of [latex]f(x)=\sin(x−π)[/latex] shifts to the right by π units, which is more than than we see in the graph of [latex]f(x)=\sin(x−\frac{π}{iv})[/latex], which shifts to the correct past [latex]\frac{π}{four}[/latex]units.

Figure 11

While C relates to the horizontal shift, D indicates the vertical shift from the midline in the general formula for a sinusoidal part. The function [latex]y=\cos(x)+D[/latex] has its midline at [latex]y=D[/latex].

Figure 12

Any value of D other than nothing shifts the graph up or down. Figure 13 compares [latex]f(x)=\sin x[/latex] with [latex]f(x)=\sin (x)+two[/latex], which is shifted 2 units up on a graph.

Effigy xiii

A General Note: Variations of Sine and Cosine Functions

Given an equation in the grade [latex]f(x)=A\sin(Bx−C)+D[/latex] or [latex]f(x)=A\cos(Bx−C)+D[/latex], [latex]\frac{C}{B}[/latex]is the phase shift and D is the vertical shift.

Instance 3: Identifying the Stage Shift of a Function

Make up one's mind the direction and magnitude of the phase shift for [latex]f(ten)=\sin(x+\frac{π}{6})−2[/latex].

Effort It

Determine the direction and magnitude of the phase shift for [latex]f(x)=3\cos(x−\frac{\pi}{2})[/latex].

Show Solution

[latex]\frac{π}{2}[/latex]; correct

Instance iv: Identifying the Vertical Shift of a Role

Decide the direction and magnitude of the vertical shift for [latex]f(x)=\cos(x)−3[/latex].

Show Solution

Let'southward begin by comparison the equation to the general class [latex]y=A\cos(Bx−C)+D[/latex]. In the given equation, [latex]D=-three[/latex], and then the shift is 3 units downward.

Try It

Determine the direction and magnitude of the vertical shift for [latex]f(x)=3\sin(x)+2[/latex].

How To: Given a sinusoidal office in the form [latex]f(x)=A\sin(Bx−C)+D[/latex], place the midline, aamplitude, flow, and phase shift.

1. Determine the amplitude equally |A|.
2. Determine the menstruum equally [latex]P=\frac{2π}{|B|}[/latex].
3. Decide the phase shift as [latex]\frac{C}{B}[/latex].
4. Determine the midline as y= D.

Instance 5: Identifying the Variations of a Sinusoidal Function from an Equation

Determine the midline, amplitude, period, and phase shift of the office [latex]y=3\sin(2x)+1[/latex].

Try It

Make up one's mind the midline, aamplitude, menstruum, and phase shift of the function [latex]y=\frac{1}{2}\cos(\frac{x}{3}−\frac{π}{3})[/latex].

Show Solution

midline: [latex]y=0[/latex]; amplitude: |A|=[latex]\frac{1}{ii}[/latex]; period: P=[latex]\frac{2π}{|B|}=6\pi[/latex]; phase shift:[latex]\frac{C}{B}=\pi[/latex]

Effort It

Case 6: Identifying the Equation for a Sinusoidal Role from a Graph

Make up one's mind the formula for the cosine function in Figure 15.

Figure 15

Try It

Decide the formula for the sine role in Figure 16.

Figure 16

Show Solution

[latex]f(ten)=\sin(10)+2[/latex]

Endeavour It

Example 7: Identifying the Equation for a Sinusoidal Role from a Graph

Determine the equation for the sinusoidal role in Figure 17.

Figure 17

Endeavor It

Write a formula for the office graphed in Figure xviii.

Figure 18

Prove Solution

ii possibilities are: [latex]y=4\sin(\frac{π}{five}ten−\frac{π}{5})+4[/latex] or [latex]y=−4sin(\frac{π}{v}x+4\frac{π}{5})+4[/latex]

Try It

Graphing Variations of y = sin x and y = cos x

Throughout this section, we have learned virtually types of variations of sine and cosine functions and used that data to write equations from graphs. Now nosotros can use the aforementioned information to create graphs from equations.

Instead of focusing on the general course equations

[latex]y=A\sin(Bx−C)+D[/latex] and [latex]y=A\cos(Bx−C)+D[/latex],

we will permit C = 0 and D = 0 and work with a simplified form of the equations in the following examples.

How To: Given the function [latex]y=Asin(Bx)[/latex], sketch its graph.

1. Identify the aamplitude,|A|.
2. Place the menses, [latex]P=\frac{2π}{|B|}[/latex].
3. Start at the origin, with the function increasing to the right if A is positive or decreasing if A is negative.
4. At [latex]ten=\frac{π}{two|B|}[/latex] at that place is a local maximum for A > 0 or a minimum for A < 0, with y = A.
5. The curve returns to the x-centrality at [latex]x=\frac{π}{|B|}[/latex].
6. There is a local minimum for A > 0 (maximum for A< 0) at [latex]x=\frac{3π}{2|B|}[/latex] with y= –A.
7. The curve returns over again to the x-axis at [latex]x=\frac{π}{2|B|}[/latex].

Example viii: Graphing a Office and Identifying the Amplitude and Menstruum

Sketch a graph of [latex]f(x)=−2\sin(\frac{πx}{2})[/latex].

Endeavour Information technology

Sketch a graph of [latex]g(ten)=−0.eight\cos(2x)[/latex]. Decide the midline, amplitude, period, and phase shift.

Show Solution

midline: y=0; amplitude: |A|=0.viii; menstruation: P=[latex]\frac{2π}{|B|}=\pi[/latex]; phase shift: [latex]\frac{C}{B}=0[/latex] or none

How To: Given a sinusoidal role with a stage shift and a vertical shift, sketch its graph.

1. Limited the office in the general form [latex]y=A\sin(Bx−C)+D[/latex] or [latex]y=A\cos(Bx−C)+D[/latex].
2. Identify the aamplitude, |A|.
3. Identify the period, [latex]P=2π|B|[/latex].
4. Identify the phase shift, [latex]\frac{C}{B}[/latex].
5. Draw the graph of [latex]f(x)=A\sin(Bx)[/latex] shifted to the right or left past [latex]\frac{C}{B}[/latex] and up or downwardly by D.

Example 9: Graphing a Transformed Sinusoid

Sketch a graph of [latex]f(10)=3\sin\left(\frac{π}{4}x−\frac{π}{4}\right)[/latex].

 Effort It

Describe a graph of [latex]m(x)=−2\cos(\frac{\pi}{3}x+\frac{\pi}{6})[/latex]. Determine the midline, aamplitude, catamenia, and stage shift.

Show Solution

[latex]\text{midline:}y=0;\text{amplitude:}|A|=two;\text{period:}\text{P}=\frac{ii\pi}{|B|}=6;\text{phase shift:}\frac{C}{B}=−\frac{1}{two}[/latex]

Attempt Information technology

Instance 10: Identifying the Properties of a Sinusoidal Function

Given [latex]y=−ii\cos\left(\frac{\pi}{2}x+\pi\right)+iii[/latex], determine the amplitude, period, phase shift, and horizontal shift. So graph the function.

Using Transformations of Sine and Cosine Functions

We can use the transformations of sine and cosine functions in numerous applications. As mentioned at the get-go of the affiliate, circular motion tin can be modeled using either the sine or cosine function.

Instance 11: Finding the Vertical Component of Circular Motion

A point rotates around a circle of radius three centered at the origin. Sketch a graph of the y-coordinate of the bespeak as a office of the angle of rotation.

Try It

What is the aamplitude of the function [latex]f(ten)=7\cos(ten)[/latex]? Sketch a graph of this role.

Show Solution

7

Example 12: Finding the Vertical Component of Round Motility

A circle with radius 3 ft is mounted with its eye 4 ft off the ground. The point closest to the footing is labeled P, as shown in Figure 23. Sketch a graph of the height higher up the ground of the bespeak P as the circle is rotated; then discover a role that gives the superlative in terms of the angle of rotation.

Figure 23

Try Information technology

A weight is attached to a spring that is so hung from a board, as shown in Effigy 25. As the spring oscillates up and down, the position y of the weight relative to the board ranges from –1 in. (at time x = 0) to –7in. (at time x = π) below the board. Assume the position of y is given as a sinusoidal function of x. Sketch a graph of the role, and and so observe a cosine role that gives the position y in terms of x.

Figure 25

Show Solution

[latex]y=3\cos(10)−four[/latex]

Example xiii: Determining a Passenger's Height on a Ferris Wheel

The London Eye is a huge Ferris wheel with a diameter of 135 meters (443 anxiety). It completes 1 rotation every thirty minutes. Riders board from a platform ii meters in a higher place the basis. Express a passenger'southward summit in a higher place footing as a function of time in minutes.

Effort It

Key Equations

Sinusoidal functions	[latex]f(10)=A\sin(Bx−C)+D[/latex]
	[latex]f(x)=A\cos(Bx−C)+D[/latex]

Key Concepts

• Periodic functions repeat later a given value. The smallest such value is the period. The basic sine and cosine functions have a period of 2π.
• The office sin x is odd, so its graph is symmetric about the origin. The function cos x is fifty-fifty, so its graph is symmetric about the y-centrality.
• The graph of a sinusoidal office has the same general shape as a sine or cosine function.
• In the general formula for a sinusoidal function, the period is [latex]\text{P}=\frac{ii\pi}{|B|}[/latex].
• In the general formula for a sinusoidal function, |A|represents aamplitude. If |A| > 1, the function is stretched, whereas if|A| < 1, the role is compressed.
• The value [latex]\frac{C}{B}[/latex] in the general formula for a sinusoidal function indicates the stage shift.
• The value D in the general formula for a sinusoidal office indicates the vertical shift from the midline.
• Combinations of variations of sinusoidal functions tin be detected from an equation.
• The equation for a sinusoidal function can be adamant from a graph.
• A function can be graphed by identifying its aamplitude and period.
• A function can also be graphed by identifying its amplitude, flow, stage shift, and horizontal shift.
• Sinusoidal functions can be used to solve existent-earth problems.

Glossary

amplitude

the vertical height of a part; the abiding A appearing in the definition of a sinusoidal function

midline

the horizontal line y= D, where D appears in the general course of a sinusoidal function

periodic function

a part f(x) that satisfies [latex]f(ten+P)=f(x)[/latex] for a specific abiding Pand whatever value of 10

phase shift

the horizontal deportation of the basic sine or cosine function; the abiding [latex]\frac{C}{B}[/latex]

sinusoidal function

whatsoever function that tin can be expressed in the form [latex]f(10)=A\sin(Bx−C)+D[/latex] or [latex]f(x)=A\cos(Bx−C)+D[/latex]

Graph Symmetric To X Axis,

Source: https://courses.lumenlearning.com/precalculus/chapter/graphs-of-the-sine-and-cosine-function/

Posted by: johnsonarefling.blogspot.com

Share this post